Radius of Convergence Calculator
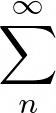
Solution


Radius of Convergence Lesson
What is Radius of Convergence?
The radius of convergence of a power series is the size of the disk where the series has absolute convergence. It can be either a positive number or infinity.
A power series is an infinite series of the form:
$$\sum\limits_{n = 0}^\infty {{c_n}{{\left( {x - a} \right)}^n}}$$
Where cn is a coefficient that varies with n and the series is a function of x with its terms varying with the nth term of the series.
Now, let's take a deeper dive into what convergence means in the context of a power series. When we add an infinite number of terms as we do with a power series, the sum of those terms will either be a finite number or infinite.
When the sum of those terms is finite, it is considered to converge absolutely. If the sum of those terms is infinite, it is considered to diverge. When we solve the radius of convergence, we are finding the value of R in |x - a| < R such that the series converges.
Why do we Learn Radius of Convergence?
Compared to humans, computers are really good at certain types of calculations but have difficulties performing other types of calculations. For example, the seemingly simple ex button commonly found on hand calculators is one that the calculator's computer cannot easily and accurately solve directly.
By learning how to find the radius of convergence, we can program an otherwise incapable computer to indirectly find the value of ex via use of a power series.
If we are evaluating ex with a large exponent, a calculator's computer has to multiply large, messy numbers by large, messy numbers many times over. Because of how computers store floating-point numbers and create round-off error, this process can take the computer very long and can give an inaccurate answer.
Luckily, the power series f(x) = xn⁄n! represents the expression ex when carried out to many terms. If we check the radius of convergence for this power series, we find that it is r = ∞ and that the interval of convergence is ∞ < x < ∞. This is great news because it means the power series will converge everywhere and can be used for ex with all possible input x values.
By programming this routine into a computer, we enable it to quickly and accurately solve for the value of ex with any value of x. This is just one example of a use for the radius of convergence, and there are many more applications that work behind the scenes inside computer software to help us every day!
Calculating the Radius of Convergence of a Power Series
There are several tests we may use to solve for radius of convergence, including the ratio test and the root test. The ratio test is simple, works often, and is used by the calculator on this page so that is what we will learn about here.
The ratio test uses a ratio of the power series and a modified n + 1 version of itself to solve for a radius of x which satisfies the convergence criteria. The ratio test formula is given as:
$$\text{Convergence when} \; L < 1, \; L = \lim_{n\to\infty} \left\lvert\frac{a_{n+1}}{a_{n}} \right\rvert$$
Where an is the power series and an + 1 is the power series with all terms n replaced with n + 1.
First, we plug each version of the power series into its respective side of the fraction inside the formula. Then, we simplify the fraction when possible.
Next, we evaluate the limit as n approaches infinity. When we plug infinity in for each instance of n, we end up with an expression that may appear unsolvable. However, we may use the standard infinite limit reduction strategies such as eliminating insignificant, non-infinite terms.
Once the limit is evaluated and reduced to its simplest form, we set it in the inequality L < 1. We will now have an inequality resembling the form of 1⁄c×|x - a| < 1.
The radius of convergence is equal to the constant c because moving it over to the right side such that |x - a| < c provides a radius of x values that satisfy the convergence criteria.
Example Problem
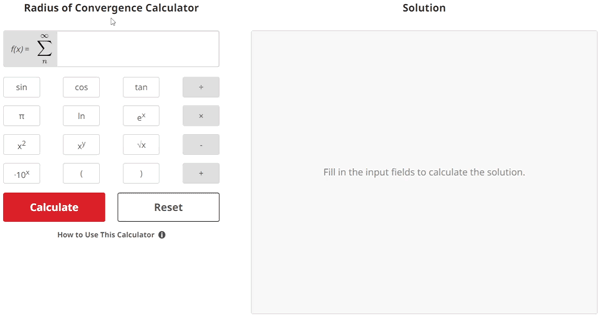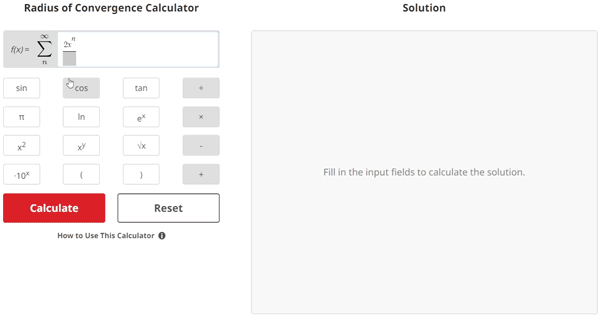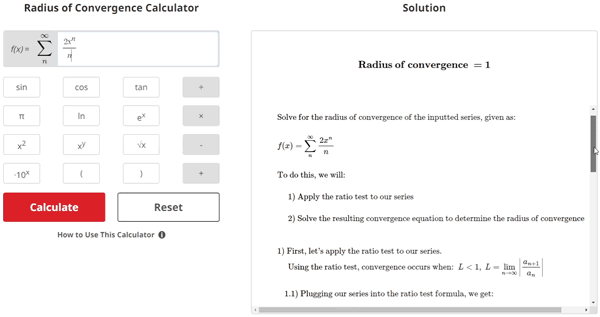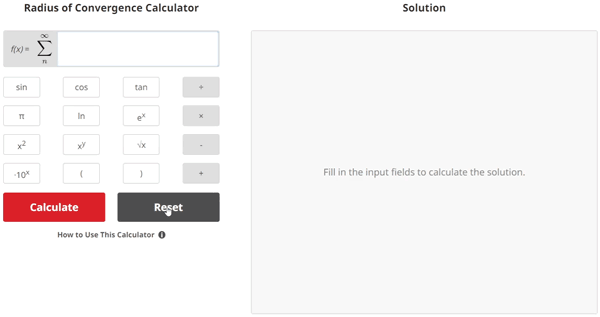
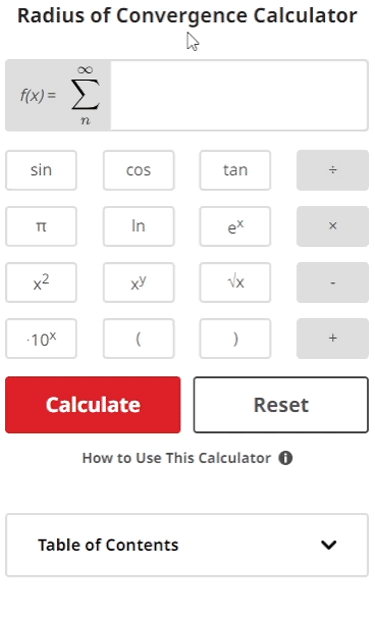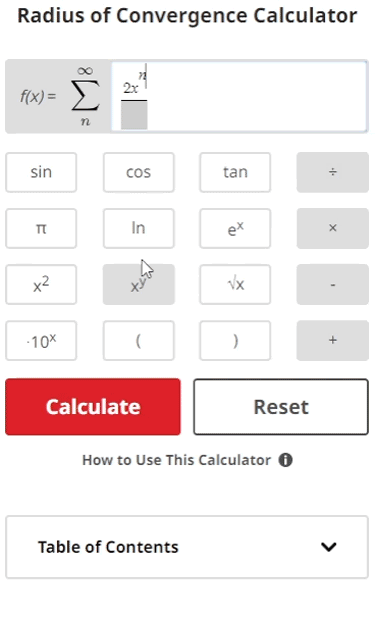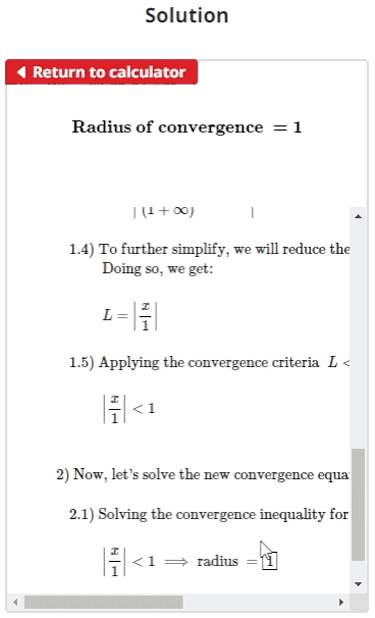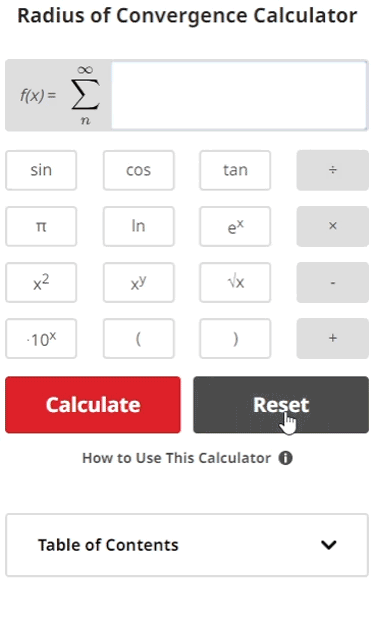
$$\begin{align} & \hspace{2ex} \text{Let's solve for the radius of convergence of the power series:} \\ \\ & \hspace{2ex} f(x) = \sum_{n}^{\infty}\frac{2x^n}{n}\\ \\ & \hspace{2ex} \text{To do this, we will:} \\ \\ & \hspace{5ex} \text{1) Apply the ratio test to our series} \\ \\ & \hspace{5ex} \text{2) Solve the resulting convergence equation to determine the radius of convergence}\\ \\ \\ & \hspace{2ex} \text{1) First, let's apply the ratio test to our series.} \\ & \hspace{5ex} \text{Using the ratio test, convergence occurs when: } \; L < 1, \; L = \lim_{n\to\infty} \left\lvert\frac{a_{n+1}}{a_{n}} \right\rvert\\ \\ & \hspace{4ex} \text{1.1) Plugging our series into the ratio test formula, we get: } \\ \\ & \hspace{9ex} a_{n} = \frac{2x^n}{n}\\ \\ & \hspace{9ex} a_{n+1} = \frac{2}{{\left(n + 1\right)}^{1}} \cdot {x}^{\left(n + 1\right)}\\ \\ & \hspace{9ex} L = \lim_{n\to\infty} \left\lvert\frac{\frac{2}{{\left(n + 1\right)}^{1}} \cdot {x}^{\left(n + 1\right)}}{\frac{2x^n}{n}}\right\rvert\\ \\ & \hspace{4ex} \text{1.2) Simplifying our ratio test equation, we get:} \\ \\ & \hspace{9ex} L = \lim_{n\to\infty} \left\lvert\frac{1}{{\left(1 + n\right)}^{1}} \cdot n \cdot x\right\rvert\\ \\ & \hspace{4ex} \text{1.3) To take the limit of } L \text{ as } \: n \: \text{ approaches } \: \infty \text{, we substitute } \: \infty \: \text{ for } \: n \text{:} \\ \\ & \hspace{9ex} L = \left\lvert\frac{1}{{\left(1 + \infty\right)}^{1}} \cdot \infty \cdot x\right\rvert\\ \\ & \hspace{4ex} \text{1.4) To further simplify, we will reduce the terms that contain infinities.} \\ & \hspace{9ex} \text{Doing so, we get:} \\ \\ & \hspace{9ex} L = \left\lvert\frac{x}{1}\right\rvert\\ \\ & \hspace{4ex} \text{1.5) Applying the convergence criteria } \; L < 1 \text{, we get:} \\ \\ & \hspace{9ex} \left\lvert\frac{x}{1}\right\rvert < 1\\ \\ \\ & \hspace{2ex} \text{2) Now, let's solve the new convergence equation to determine the radius of convergence.}\\ \\ & \hspace{4ex} \text{2.1) Solving the convergence inequality for the radius of convergence, we get:} \\ \\ & \hspace{9ex} \left\lvert\frac{x}{1}\right\rvert < 1 \; \Longrightarrow \; \text{radius } = \boxed{1} \\ \\ & \hspace{4ex} \boxed{\text{Radius of convergence } = \boxed{1}}\\ & \end{align}$$
How the Calculator Works
The calculator on this page is written in three common web frontend languages: HTML, CSS, and JavaScript (JS). It also utilizes a JS-native computer algebra system (CAS) which performs some algebraic steps during the computation process. Because the calculator is powered by JS code, it runs entirely inside your browser's built-in JS engine and provides instant solutions and steps (no page reload needed).
When you click the "calculate" button, the solving routine is called and progresses through several symbolic operations. These operations mirror the ratio test's steps. The routine also saves the state of the limit/expression throughout the process. These "states" are used to build the solution steps which mirror that of a ratio test.
Once a final answer is calculated, it is printed in the solution box. The solution steps are printed below the answer when a user with access is logged in. If the calculator runs into an error during computation, an error message will be displayed instead of the answer and steps.